Why and How to Find a Central Angle?
Introduction to Central Angles in Civil Engineering
Civil engineers are professionals responsible for designing and constructing vital structures like airports, bridges, waterways, and transportation systems. Among the various fields within civil engineering, roadway engineering stands out as particularly significant.
Determining the Central Angle for Roadway Curves
During roadway construction, engineers often encounter the need to design curved roads – a process where determining the central angle plays a crucial role. Consider this curved road segment, known as a circular curve because it can be modeled by a circle with radius R.

When engineers design such curves, they must calculate the degree of curvature, which represents the sharpness of the road’s curve. Interestingly, calculating the degree of curvature is essentially the same as finding central angle of a circle, a concept familiar from math class!
Calculating the Central Angle for a Road Curve
The curved part of the road has a radius of 90 feet, and the length of the arc is 100 feet:
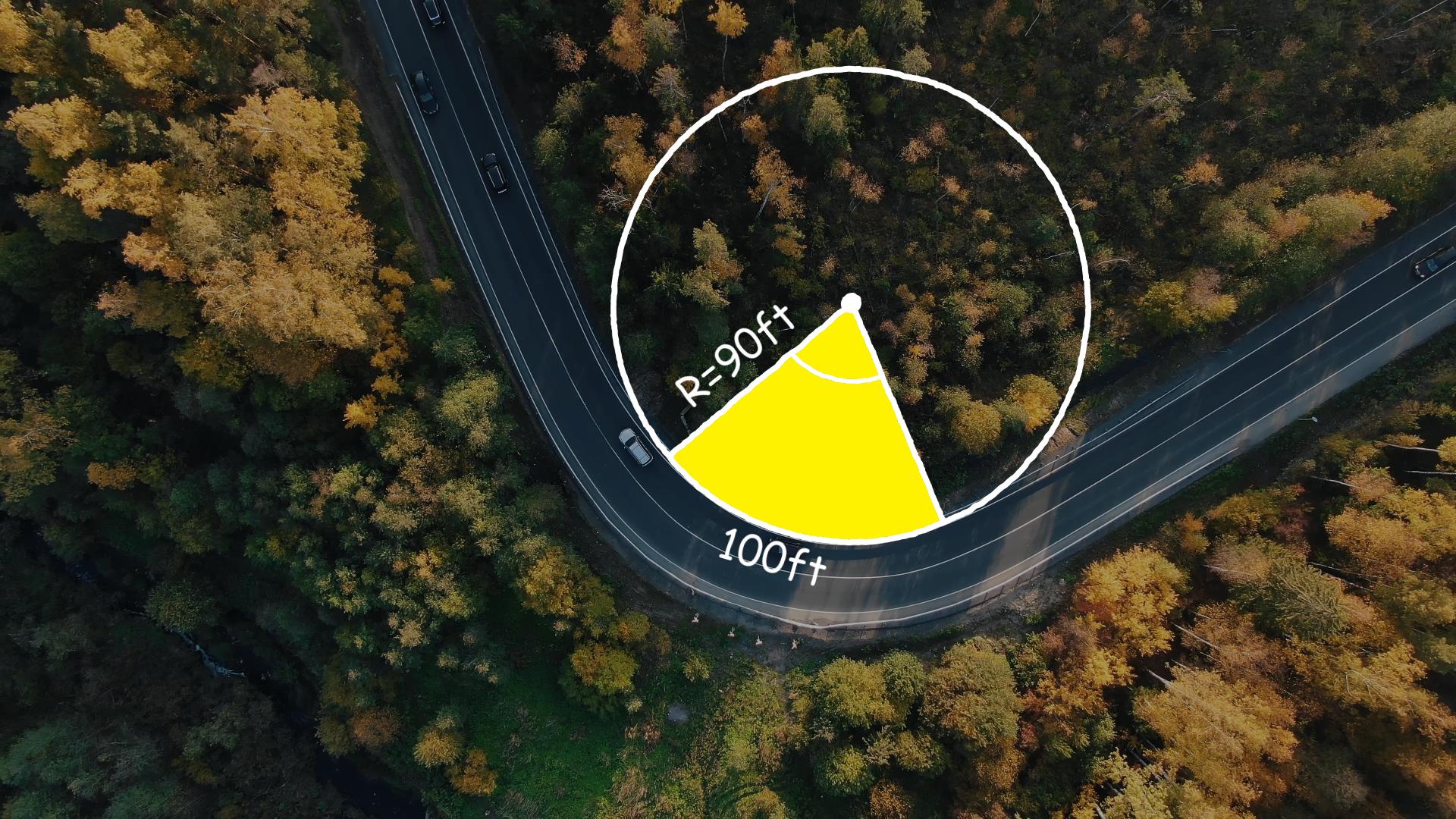
First, let’s determine the circumference length: Using the formula for circumference length, which is \(2\pi R\), our circumference equals:
\(2 \times 3.14 \times 90 = 565.2\) ft.
If the length of the arc is 100 feet, we can set up a simple proportion. In this proportion, 360 degrees corresponds to a length of 565.2 feet. So, we need to find out how many degrees, represented by x, would be equivalent to an arc length of 100 feet:
\(\frac{360}{565.2} = \frac{x}{100}\).
Solving this proportion gives us approximately 63.7 degrees. This value represents the central angle, or as a civil engineer would say, the degree of curvature of this road segment.
Significance of Finding Central Angles
Civil engineering standards and guidelines often contain specific regulations concerning various road curvatures. If a road curvature exceeds a certain degree, local laws may require lowering speed limits on a particular curve, installing guardrails, or implementing additional safety measures.
Conclusion:
In conclusion, understanding how to find the central angle (or degree of curvature) is essential in civil engineering. These calculations not only facilitate the design of safer and more efficient roadways but also ensure compliance with engineering standards and regulations. By applying mathematical principles to real-world scenarios, civil engineers play a vital role in creating and maintaining infrastructure that promotes safety and enhances the quality of life for everyone.
References:
We have utilized several sources to compile this article:
- General article on Wikipedia about the degree of curvature;
- Survey manual issued by the Wyoming Department of Transportation (Appendix D);
- Hauer, E. (1999). Safety and the choice of degree of curve. Transportation Research Record, 1665(1), 22-27.
Video Version:
The video version of this article expands upon the concepts discussed here, providing additional examples of central angle calculations. It demonstrates the calculation for both sharp and gentle curves, allowing for a comparison between them, and also explains the importance of unit conversions. Additionally, it illustrates how to address sharp curves, particularly in the context of railroads. With enhanced animations and easy-to-follow explanations, the video version offers an engaging learning experience. Enjoy the preview below, or subscribe to access all our full videos.
Further reading:
Curious about how other math concepts discussed here are applied in real life? In this article, we’ve explored concepts such as circumference, angles, and proportions. We have more articles available, where we explore how these concepts are utilized in real-world scenarios:
- The article “Circumference: A Real-Life Example (from Microbiology)” explains how the circumference and area of a circle are helpful in microbiology;
- The article “Alternate and Adjacent Angles: A Real-Life Example (from Architecture)” explains why the calculation of angles is very important in modern architecture and how it helps integrate solar panels into buildings;
- Finally, the article “How to Write Inequalities: A Real-life Example (from Social Media)” explains how calculating ratios and proportions helps to make the Internet safer.
