Introduction
What is an arithmetic sequence?
An arithmetic sequence is a set of numbers where the difference between consecutive terms is always the same. This difference, called the common difference, determines whether the sequence increases or decreases.
For example:
- 2, 5, 8, 11 is an arithmetic sequence with a common difference of 3, so it increases.
- 10, 7, 4, 1 has a common difference of -3, so it decreases.
Arithmetic sequences grow or shrink at a steady rate, making them useful in many real-world applications.
Real-life applications of arithmetic sequences
Arithmetic sequences are used in many real-life situations, from construction and finance to automotive design and mechanical engineering. In this article, we’ll focus on a detailed example from mechanical engineering.
Arithmetic sequence in mechanical engineering
Mechanical design of bicycle gears
You’ve probably ridden a bicycle and shifted gears. When you shift, the chain moves from one sprocket to another, depending on whether you’re riding on flat terrain or climbing hills. But how do mechanical engineers decide how many teeth each sprocket in a cassette should have? Surprisingly, one method they use to determine the number of teeth is an arithmetic sequence.
Arithmetic sequence defines the difference
The basic idea is that the number of teeth on each sprocket differs from the previous one by a constant amount. For example, take a look at the 6-sprocket cassette shown below:
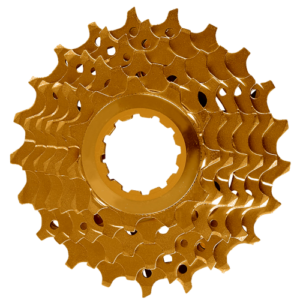
The number of teeth on the sprockets (from 1st gear to 6th gear) is: 21, 19, 17, 15, 13, and 11 teeth. You may have already noticed that the number of teeth forms a decreasing arithmetic sequence.
Connection with linear function
In 8th grade, you’ll learn about linear functions. In fact, arithmetic sequences are closely related to linear functions. To show this, let’s plot our sequence on a Cartesian coordinate plane, where the x-axis represents the gear number and the y-axis represents the number of teeth on each sprocket:
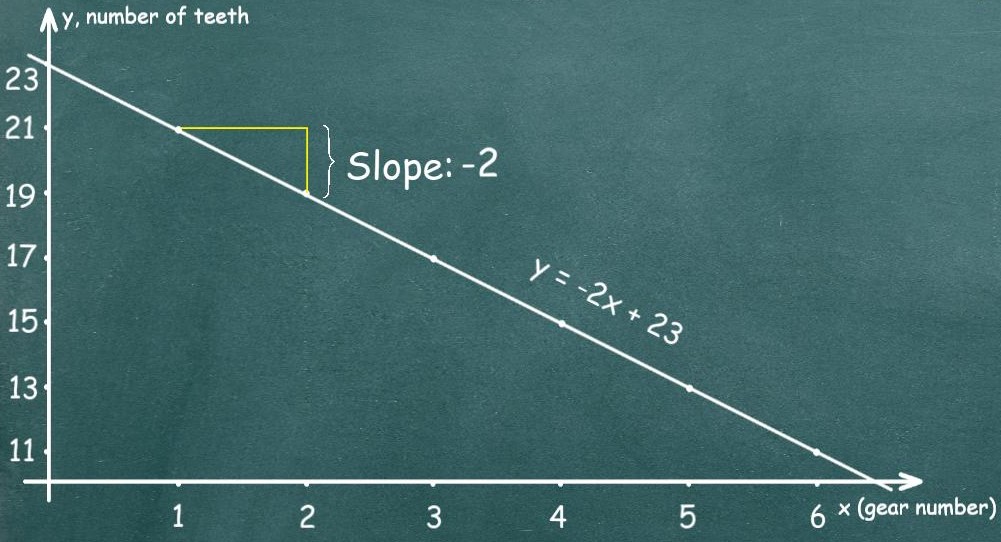
You’ll see that the arithmetic sequence follows the linear function y = -2x + 23, where -2 is the slope of the function, which matches the common difference of our arithmetic sequence.
Want more? Watch the arithmetic sequences video!
We also have a video version where this application of arithmetic sequences is explained in an easy-to-follow, engaging, and animated format. Enjoy the preview below, and consider subscribing to access all of our full videos.
Conclusion
Using an arithmetic sequence to define the number of teeth on each consecutive sprocket is the simplest approach, and it works well for basic bicycle drivetrain systems. However, for more complex systems, like those on mountain bikes, a different method, such as a geometric sequence, might be a better fit. That’s why, if you’re interested in mechanical engineering, understanding patterns and sequences is key to becoming a skilled specialist.
References:
To ensure the accuracy of this article, we consulted the following source, which explains how mathematical sequences are used in designing mechanical gears:
Further reading:
We also have a complementary article explaining the application of geometric sequences to the same task of designing bicycle cassettes: Geometric Sequence: A Real-Life Example (from Mechanical Engineering).