Pythagorean Theorem Converse in Construction and Carpentry
Introduction
From your math classes, you’re familiar with the Pythagorean theorem stating that, in a right triangle, the square of the hypotenuse equals the sum of the squares of the legs. Another important concept is the Pythagorean theorem converse: if the square of the longest side of a triangle is equal to the sum of the squares of the other two sides, then the triangle is a right triangle. Did you know that this rule is actively used in various DIY projects, especially those related to construction and carpentry? Let’s explore a specific example!
Example:
Many people enjoy creating wonderful things and building their homes themselves. Constructing a solid foundation is a crucial step for any building; it must be level and square. But how do we ensure that all angles are precisely 90 degrees? This is where the Pythagorean theorem converse comes to the rescue!
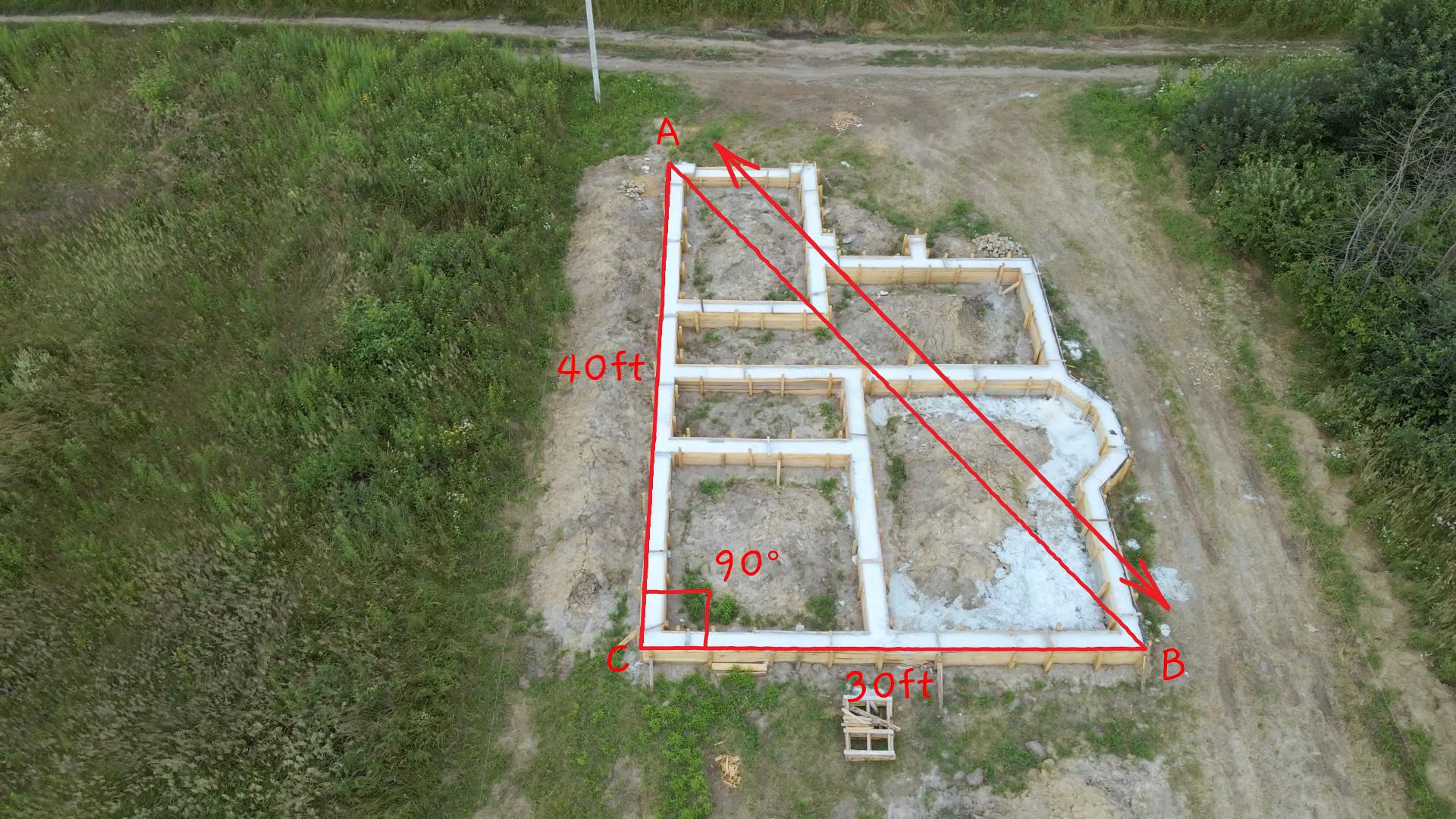
Take a look at the picture above. This foundation has dimensions of 30 by 40 feet, allowing us to create a triangle ABC with short sides of 30 and 40 feet. Applying the converse of the Pythagorean theorem gives us:
30² + 40² = 2500.
To guarantee that angle BCA is 90 degrees, we measure the longest side of this triangle (side AB). If our angle is 90 degrees, then this side must be:
√2500 = 50 feet long.
A shorter side means the angle is less than 90 degrees (the triangle, and thus the foundation, is acute). A longer side means the angle is over 90 degrees (the triangle, and consequently the foundation, is obtuse).
This simple technique is widely used by DIY carpenters and helps create right angles wherever needed: in foundations, walls, roofs, and more.
Conclusions:
The example you’ve just seen is a practical demonstration of how math knowledge is valuable in real life. Remember, this is just one instance showcasing the usefulness of the Pythagorean theorem! In fact, the Pythagorean theorem and its converse are helpful whenever ensuring that an angle is precisely 90° is essential. This is not only applicable in carpentry but also in construction and civil engineering.
References:
This time, we include a link to a video titled ‘Framing Floors and Stairs with Larry Haun.’ In this video, you can witness firsthand how Larry Haun (1931 – 2011), one of the world’s finest carpenters, checks the foundation using the converse of the Pythagorean theorem. At the 0:50 mark of the video, Larry Haun demonstrates a 6-8-10 check to ensure that the angle is 90 degrees. He marks the 6 and 8 feet sides of a triangle and then verifies if the longest side (hypotenuse) equals 10 ft:
√(6² + 8²) = 10 ft.
Check the video and see for yourself:
Video version:
In the video version of this article, we’ve also incorporated another example of how the inverse of the Pythagorean theorem proves useful in gardening! Watch the preview of this easy-to-follow animated video below or subscribe for access to the full version. (Why subscribe?)
